Draw a Free Body Diagram for the Weight Lifter

Learning Objectives

Past the end of the section, you will exist able to:

• Explain the rules for cartoon a gratuitous-body diagram
• Construct free-torso diagrams for dissimilar situations

The first pace in describing and analyzing most phenomena in physics involves the conscientious drawing of a complimentary-body diagram. Gratis-body diagrams take been used in examples throughout this chapter. Remember that a complimentary-trunk diagram must only include the external forces acting on the body of involvement. Once we take drawn an authentic complimentary-body diagram, we can apply Newton's first law if the body is in equilibrium (balanced forces; that is, [latex] {F}_{\text{net}}=0 [/latex]) or Newton'southward second law if the body is accelerating (unbalanced force; that is, [latex] {F}_{\text{net}}\ne 0 [/latex]).

In Forces, we gave a brief problem-solving strategy to aid you lot understand costless-body diagrams. Hither, we add some details to the strategy that will help you lot in constructing these diagrams.

Trouble-Solving Strategy: Amalgam Free-Torso Diagrams

Observe the post-obit rules when constructing a gratuitous-body diagram:

1. Draw the object under consideration; it does non have to be artistic. At start, you may want to describe a circle effectually the object of interest to exist sure y'all focus on labeling the forces interim on the object. If you are treating the object as a particle (no size or shape and no rotation), represent the object as a point. We often place this point at the origin of an xy-coordinate system.
2. Include all forces that deed on the object, representing these forces as vectors. Consider the types of forces described in Common Forces—normal force, friction, tension, and spring force—as well equally weight and applied forcefulness. Do non include the internet forcefulness on the object. With the exception of gravity, all of the forces nosotros have discussed require direct contact with the object. However, forces that the object exerts on its surroundings must not be included. We never include both forces of an action-reaction pair.
3. Catechumen the free-body diagram into a more detailed diagram showing the ten– and y-components of a given force (this is often helpful when solving a problem using Newton's first or second law). In this example, place a squiggly line through the original vector to show that it is no longer in play—it has been replaced past its 10– and y-components.
4. If there are two or more objects, or bodies, in the trouble, draw a separate costless-torso diagram for each object.

Note: If there is dispatch, we do non directly include it in the free-body diagram; however, it may aid to point acceleration exterior the gratuitous-body diagram. You can characterization information technology in a dissimilar colour to indicate that it is separate from the free-body diagram.

Let'south utilise the trouble-solving strategy in drawing a free-body diagram for a sled. In (Figure)(a), a sled is pulled by force P at an bending of [latex] thirty\text{°} [/latex]. In part (b), nosotros show a free-body diagram for this state of affairs, as described by steps 1 and 2 of the problem-solving strategy. In part (c), nosotros prove all forces in terms of their x– and y-components, in keeping with step three.

Figure 5.31 (a) A moving sled is shown every bit (b) a free-body diagram and (c) a costless-trunk diagram with force components.

Case

Two Blocks on an Inclined Aeroplane

Construct the gratis-body diagram for object A and object B in (Figure).

Strategy

We follow the four steps listed in the problem-solving strategy.

Solution

We beginning past creating a diagram for the first object of involvement. In (Figure)(a), object A is isolated (circled) and represented by a dot.

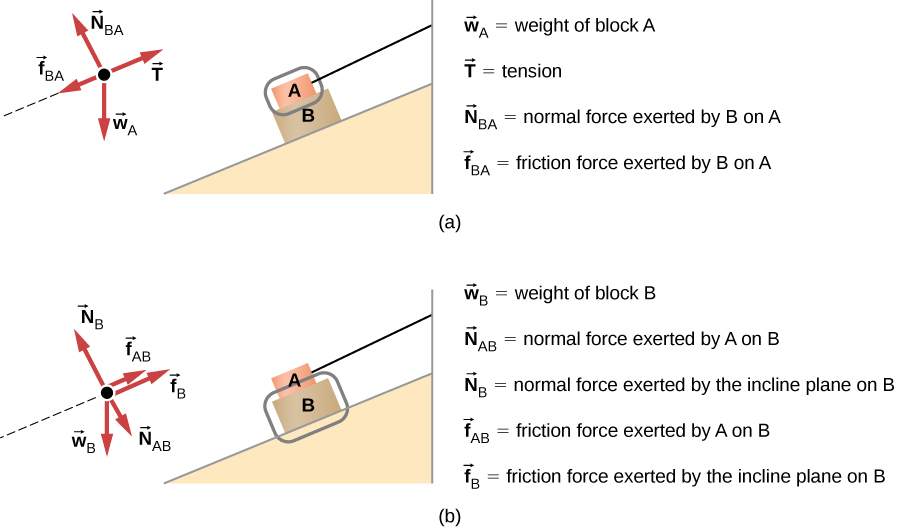
Figure 5.32 (a) The free-body diagram for isolated object A. (b) The gratis-body diagram for isolated object B. Comparing the two drawings, we come across that friction acts in the opposite direction in the ii figures. Because object A experiences a force that tends to pull information technology to the right, friction must act to the left. Because object B experiences a component of its weight that pulls it to the left, down the incline, the friction force must oppose information technology and act up the ramp. Friction always acts opposite the intended direction of movement.

We at present include any force that acts on the body. Here, no practical forcefulness is nowadays. The weight of the object acts as a force pointing vertically downward, and the presence of the cord indicates a force of tension pointing away from the object. Object A has one interface and hence experiences a normal force, directed away from the interface. The source of this forcefulness is object B, and this normal strength is labeled accordingly. Since object B has a tendency to slide down, object A has a tendency to slide upwards with respect to the interface, so the friction [latex] {f}_{\text{BA}} [/latex] is directed downwards parallel to the inclined plane.

Every bit noted in footstep iv of the trouble-solving strategy, we then construct the costless-body diagram in (Effigy)(b) using the same approach. Object B experiences two normal forces and two friction forces due to the presence of ii contact surfaces. The interface with the inclined plane exerts external forces of [latex] {Northward}_{\text{B}} [/latex] and [latex] {f}_{\text{B}} [/latex], and the interface with object B exerts the normal strength [latex] {N}_{\text{AB}} [/latex] and friction [latex] {f}_{\text{AB}} [/latex]; [latex] {Northward}_{\text{AB}} [/latex] is directed away from object B, and [latex] {f}_{\text{AB}} [/latex] is opposing the tendency of the relative motion of object B with respect to object A.

Significance

The object under consideration in each function of this problem was circled in gray. When you are first learning how to describe free-trunk diagrams, you will find it helpful to circle the object before deciding what forces are acting on that particular object. This focuses your attention, preventing you from because forces that are not acting on the body.

Example

Two Blocks in Contact

A strength is applied to 2 blocks in contact, as shown.

Strategy

Depict a free-body diagram for each cake. Be sure to consider Newton's 3rd law at the interface where the two blocks touch.

Solution

Significance[latex] {\overset{\to }{A}}_{21} [/latex] is the activity force of cake 2 on block 1. [latex] {\overset{\to }{A}}_{12} [/latex] is the reaction force of block 1 on block two. We apply these free-body diagrams in Applications of Newton's Laws.

Instance

Cake on the Table (Coupled Blocks)

A block rests on the tabular array, as shown. A light rope is attached to it and runs over a pulley. The other end of the rope is attached to a second block. The two blocks are said to be coupled. Block [latex] {m}_{2} [/latex] exerts a force due to its weight, which causes the organisation (ii blocks and a cord) to accelerate.

Strategy

We assume that the string has no mass so that we do not have to consider it as a split object. Draw a free-body diagram for each block.

Solution

Significance

Each block accelerates (notice the labels shown for [latex] {\overset{\to }{a}}_{1} [/latex] and [latex] {\overset{\to }{a}}_{ii} [/latex]); notwithstanding, assuming the string remains taut, they accelerate at the same rate. Thus, we have [latex] {\overset{\to }{a}}_{ane}={\overset{\to }{a}}_{2} [/latex]. If we were to go on solving the problem, we could simply call the acceleration [latex] \overset{\to }{a} [/latex]. Also, we apply two gratis-body diagrams because we are usually finding tension T, which may require us to apply a organisation of ii equations in this type of problem. The tension is the same on both [latex] {m}_{ane}\,\text{and}\,{m}_{2} [/latex].

Check Your Understanding

(a) Draw the free-body diagram for the state of affairs shown. (b) Redraw it showing components; apply 10-axes parallel to the two ramps.

Show Solution

Effigy a shows a gratis body diagram of an object on a line that slopes down to the right. Arrow T from the object points right and up, parallel to the slope. Pointer N1 points left and up, perpendicular to the gradient. Arrow w1 points vertically down. Arrow w1x points left and down, parallel to the slope. Arrow w1y points right and down, perpendicular to the gradient. Figure b shows a free torso diagram of an object on a line that slopes down to the left. Arrow N2 from the object points correct and up, perpendicular to the slope. Arrow T points left and up, parallel to the slope. Pointer w2 points vertically downwardly. Arrow w2y points left and down, perpendicular to the slope. Arrow w2x points right and down, parallel to the gradient.

View this simulation to predict, qualitatively, how an external force will touch the speed and direction of an object's move. Explain the effects with the help of a costless-body diagram. Employ costless-torso diagrams to describe position, velocity, acceleration, and force graphs, and vice versa. Explain how the graphs relate to one another. Given a scenario or a graph, sketch all four graphs.

Summary

• To draw a gratis-body diagram, we draw the object of interest, depict all forces acting on that object, and resolve all force vectors into 10– and y-components. We must draw a separate free-body diagram for each object in the problem.
• A costless-trunk diagram is a useful means of describing and analyzing all the forces that act on a body to decide equilibrium according to Newton's first law or acceleration according to Newton'south 2nd law.

Fundamental Equations

Net external strength	[latex] {\overset{\to }{F}}_{\text{net}}=\sum \overset{\to }{F}={\overset{\to }{F}}_{ane}+{\overset{\to }{F}}_{2}+\text{⋯} [/latex]
Newton'southward get-go law	[latex] \overset{\to }{5}=\,\text{abiding when}\,{\overset{\to }{F}}_{\text{internet}}=\overset{\to }{0}\,\text{N} [/latex]
Newton's second law, vector form	[latex] {\overset{\to }{F}}_{\text{net}}=\sum \overset{\to }{F}=yard\overset{\to }{a} [/latex]
Newton's second constabulary, scalar form	[latex] {F}_{\text{internet}}=ma [/latex]
Newton'southward second law, component grade	[latex] \sum {\overset{\to }{F}}_{x}=m{\overset{\to }{a}}_{x}\text{,}\,\sum {\overset{\to }{F}}_{y}=yard{\overset{\to }{a}}_{y},\,\text{and}\,\sum {\overset{\to }{F}}_{z}=m{\overset{\to }{a}}_{z}. [/latex]
Newton'due south second law, momentum form	[latex] {\overset{\to }{F}}_{\text{internet}}=\frac{d\overset{\to }{p}}{dt} [/latex]
Definition of weight, vector form	[latex] \overset{\to }{due west}=m\overset{\to }{g} [/latex]
Definition of weight, scalar grade	[latex] westward=mg [/latex]
Newton'southward third law	[latex] {\overset{\to }{F}}_{\text{AB}}=\text{−}{\overset{\to }{F}}_{\text{BA}} [/latex]
Normal strength on an object resting on a horizontal surface, vector form	[latex] \overset{\to }{N}=\text{−}thousand\overset{\to }{g} [/latex]
Normal force on an object resting on a horizontal surface, scalar class	[latex] N=mg [/latex]
Normal forcefulness on an object resting on an inclined airplane, scalar course	[latex] North=mg\text{cos}\,\theta [/latex]
Tension in a cable supporting an object of mass m at residuum, scalar form	[latex] T=w=mg [/latex]

Conceptual Questions

In completing the solution for a trouble involving forces, what do nosotros do later on constructing the costless-body diagram? That is, what do we apply?

If a book is located on a table, how many forces should be shown in a free-body diagram of the book? Describe them.

Show Solution

Ii forces of different types: weight acting downward and normal force interim upwards

If the volume in the previous question is in free autumn, how many forces should exist shown in a free-trunk diagram of the volume? Describe them.

Bug

A ball of mass thou hangs at residue, suspended past a string. (a) Sketch all forces. (b) Describe the free-torso diagram for the ball.

A auto moves along a horizontal route. Describe a free-torso diagram; be sure to include the friction of the route that opposes the forward motion of the car.

Show Solution

A runner pushes confronting the track, as shown. (a) Provide a free-body diagram showing all the forces on the runner. (Hint: Place all forces at the eye of his trunk, and include his weight.) (b) Give a revised diagram showing the xy-component form.

The traffic low-cal hangs from the cables equally shown. Draw a free-torso diagram on a coordinate plane for this situation.

Show Solution

Boosted Bug

Two small forces, [latex] {\overset{\to }{F}}_{1}=-ii.40\hat{i}-6.10t\hat{j} [/latex] N and [latex] {\overset{\to }{F}}_{ii}=8.l\hat{i}-9.seventy\hat{j} [/latex] N, are exerted on a rogue asteroid past a pair of infinite tractors. (a) Find the net force. (b) What are the magnitude and management of the net force? (c) If the mass of the asteroid is 125 kg, what acceleration does it experience (in vector course)? (d) What are the magnitude and direction of the acceleration?

2 forces of 25 and 45 Northward act on an object. Their directions differ past [latex] seventy\text{°} [/latex]. The resulting dispatch has magnitude of [latex] 10.0\,{\text{m/s}}^{2}. [/latex] What is the mass of the torso?

A force of 1600 North acts parallel to a ramp to push a 300-kg pianoforte into a moving van. The ramp is inclined at [latex] 20\text{°} [/latex]. (a) What is the acceleration of the pianoforte up the ramp? (b) What is the velocity of the pianoforte when it reaches the top if the ramp is 4.0 m long and the piano starts from remainder?

Depict a free-trunk diagram of a diver who has entered the h2o, moved downward, and is acted on by an up force due to the h2o which balances the weight (that is, the diver is suspended).

Show Solution

For a swimmer who has but jumped off a diving board, presume air resistance is negligible. The swimmer has a mass of 80.0 kg and jumps off a board ten.0 yard above the water. Three seconds after inbound the water, her downward motion is stopped. What boilerplate upward force did the water exert on her?

(a) Find an equation to determine the magnitude of the net force required to stop a car of mass grand, given that the initial speed of the automobile is [latex] {v}_{0} [/latex] and the stopping distance is x. (b) Find the magnitude of the internet forcefulness if the mass of the automobile is 1050 kg, the initial speed is xl.0 km/h, and the stopping distance is 25.0 m.

Show Solution

a. [latex] {F}_{\text{internet}}=\frac{m({five}^{2}-{v}_{0}{}^{2})}{2x} [/latex]; b. 2590 N

A sailboat has a mass of [latex] 1.l\,×\,{x}^{3} [/latex] kg and is acted on past a force of [latex] ii.00\,×\,{ten}^{3} [/latex] Northward toward the e, while the current of air acts behind the sails with a force of [latex] 3.00\,×\,{x}^{3} [/latex] N in a management [latex] 45\text{°} [/latex] due north of east. Find the magnitude and direction of the resulting acceleration.

Find the dispatch of the trunk of mass ten.0 kg shown beneath.

Show Reply

[latex] \begin{assortment}{cc} {\overset{\to }{F}}_{\text{cyberspace}}=four.05\hat{i}+12.0\chapeau{j}\text{Due north}\hfill \\ {\overset{\to }{F}}_{\text{net}}=m\overset{\to }{a}⇒\overset{\to }{a}=0.405\hat{i}+1.20\chapeau{j}\,{\text{k/s}}^{2}\hfill \finish{array} [/latex]

A body of mass 2.0 kg is moving along the x-axis with a speed of 3.0 m/due south at the instant represented below. (a) What is the dispatch of the body? (b) What is the torso'southward velocity x.0 s subsequently? (c) What is its displacement after 10.0 s?

Strength [latex] {\overset{\to }{F}}_{\text{B}} [/latex] has twice the magnitude of force [latex] {\overset{\to }{F}}_{\text{A}}. [/latex] Observe the management in which the particle accelerates in this effigy.

Shown below is a body of mass 1.0 kg nether the influence of the forces [latex] {\overset{\to }{F}}_{A} [/latex], [latex] {\overset{\to }{F}}_{B} [/latex], and [latex] one thousand\overset{\to }{g} [/latex]. If the body accelerates to the left at [latex] 20\,{\text{1000/s}}^{2} [/latex], what are [latex] {\overset{\to }{F}}_{A} [/latex] and [latex] {\overset{\to }{F}}_{B} [/latex]?

A force acts on a motorcar of mass k so that the speed v of the car increases with position ten as [latex] v=k{x}^{2} [/latex], where chiliad is constant and all quantities are in SI units. Notice the strength acting on the car every bit a function of position.

Show Solution

[latex] F=2kmx [/latex]; First, take the derivative of the velocity role to obtain [latex] a=2kx [/latex]. And then apply Newton's 2nd law [latex] F=ma=grand(2kx)=2kmx [/latex].

A seven.0-N force parallel to an incline is practical to a ane.0-kg crate. The ramp is tilted at [latex] twenty\text{°} [/latex] and is frictionless. (a) What is the dispatch of the crate? (b) If all other weather are the same but the ramp has a friction force of 1.9 N, what is the dispatch?

Two boxes, A and B, are at rest. Box A is on level ground, while box B rests on an inclined plane tilted at bending [latex] \theta [/latex] with the horizontal. (a) Write expressions for the normal force interim on each block. (b) Compare the ii forces; that is, tell which one is larger or whether they are equal in magnitude. (c) If the angle of incline is [latex] x\text{°} [/latex], which force is greater?

Prove Solution

a. For box A, [latex] {Northward}_{\text{A}}=mg [/latex] and [latex] {North}_{\text{B}}=mg\,\text{cos}\,\theta [/latex]; b. [latex] {Due north}_{\text{A}}>{Northward}_{\text{B}} [/latex] because for [latex] \theta <90\text{°} [/latex], [latex] \text{cos}\,\theta <i [/latex]; c. [latex] {Northward}_{\text{A}}>{N}_{\text{B}} [/latex] when [latex] \theta =10\text{°} [/latex]

A mass of 250.0 m is suspended from a spring hanging vertically. The spring stretches 6.00 cm. How much will the bound stretch if the suspended mass is 530.0 one thousand?

As shown beneath, ii identical springs, each with the bound constant 20 N/thou, back up a xv.0-N weight. (a) What is the tension in spring A? (b) What is the amount of stretch of spring A from the balance position?

Bear witness Solution

a. 8.66 Due north; b. 0.433 m

Shown below is a 30.0-kg block resting on a frictionless ramp inclined at [latex] 60\text{°} [/latex] to the horizontal. The cake is held by a bound that is stretched 5.0 cm. What is the force constant of the spring?

In building a firm, carpenters use nails from a big box. The box is suspended from a spring twice during the day to mensurate the usage of nails. At the outset of the day, the spring stretches 50 cm. At the end of the day, the spring stretches 30 cm. What fraction or per centum of the nails have been used?

Prove Solution

0.forty or 40%

A forcefulness is applied to a block to motility information technology upwardly a [latex] xxx\text{°} [/latex] incline. The incline is frictionless. If [latex] F=65.0\,\text{N} [/latex] and [latex] M=5.00\,\text{kg} [/latex], what is the magnitude of the acceleration of the cake?

Two forces are applied to a 5.0-kg object, and it accelerates at a rate of [latex] 2.0\,{\text{one thousand/s}}^{2} [/latex] in the positive y-management. If one of the forces acts in the positive x-direction with magnitude 12.0 N, discover the magnitude of the other force.

The block on the correct shown below has more mass than the block on the left ([latex] {thou}_{two}>{grand}_{1} [/latex]). Describe gratis-body diagrams for each block.

Challenge Problems

If two tugboats pull on a disabled vessel, as shown here in an overhead view, the disabled vessel volition be pulled along the direction indicated by the result of the exerted forces. (a) Draw a free-body diagram for the vessel. Assume no friction or drag forces affect the vessel. (b) Did you include all forces in the overhead view in your free-body diagram? Why or why non?

A 10.0-kg object is initially moving due east at fifteen.0 m/south. Then a force acts on it for 2.00 due south, after which it moves northwest, also at xv.0 m/s. What are the magnitude and direction of the average force that acted on the object over the 2.00-s interval?

On June 25, 1983, shot-doodle Udo Beyer of Eastward Germany threw the 7.26-kg shot 22.22 m, which at that time was a world record. (a) If the shot was released at a height of 2.20 g with a projection angle of [latex] 45.0\text{°} [/latex], what was its initial velocity? (b) If while in Beyer'southward hand the shot was accelerated uniformly over a distance of 1.20 k, what was the net strength on it?

Show Solution

a. 14.1 k/s; b. 601 N

A body of mass m moves in a horizontal management such that at time t its position is given by [latex] x(t)=a{t}^{4}+b{t}^{three}+ct, [/latex] where a, b, and c are constants. (a) What is the acceleration of the body? (b) What is the fourth dimension-dependent forcefulness interim on the torso?

A trunk of mass m has initial velocity [latex] {v}_{0} [/latex] in the positive ten-direction. It is acted on by a constant forcefulness F for time t until the velocity becomes zero; the force continues to act on the body until its velocity becomes [latex] \text{−}{v}_{0} [/latex] in the same amount of time. Write an expression for the full distance the body travels in terms of the variables indicated.

Show Solution

[latex] \frac{F}{m}{t}^{ii} [/latex]

The velocities of a iii.0-kg object at [latex] t=vi.0\,\text{s} [/latex] and [latex] t=8.0\,\text{south} [/latex] are [latex] (iii.0\chapeau{i}-6.0\hat{j}+4.0\lid{k})\,\text{g/southward} [/latex] and [latex] (-2.0\hat{i}+4.0\hat{thou})\,\text{m/s} [/latex], respectively. If the object is moving at constant acceleration, what is the force acting on it?

A 120-kg astronaut is riding in a rocket sled that is sliding forth an inclined plane. The sled has a horizontal component of dispatch of [latex] 5.0\,\text{chiliad}\text{/}{\text{south}}^{2} [/latex] and a down component of [latex] iii.8\,\text{chiliad}\text{/}{\text{s}}^{2} [/latex]. Calculate the magnitude of the strength on the rider by the sled. (Hint: Recall that gravitational acceleration must be considered.)

Two forces are acting on a 5.0-kg object that moves with dispatch [latex] two.0\,{\text{grand/southward}}^{ii} [/latex] in the positive y-management. If one of the forces acts in the positive x-direction and has magnitude of 12 N, what is the magnitude of the other forcefulness?

Suppose that yous are viewing a soccer game from a helicopter above the playing field. Two soccer players simultaneously kick a stationary soccer ball on the flat field; the soccer ball has mass 0.420 kg. The start histrion kicks with force 162 N at [latex] ix.0\text{°} [/latex] due north of west. At the aforementioned instant, the second player kicks with force 215 North at [latex] 15\text{°} [/latex] east of south. Observe the acceleration of the ball in [latex] \chapeau{i} [/latex] and [latex] \lid{j} [/latex] form.

Show Solution

[latex] [/latex][latex] \overset{\to }{a}=-248\hat{i}-433\chapeau{j}\text{1000}\text{/}{\text{s}}^{ii} [/latex]

A 10.0-kg mass hangs from a spring that has the spring constant 535 N/m. Find the position of the finish of the jump away from its rest position. (Use [latex] m=9.80\,{\text{m/s}}^{2} [/latex].)

A 0.0502-kg pair of fuzzy dice is attached to the rearview mirror of a car by a short cord. The car accelerates at constant charge per unit, and the dice hang at an bending of [latex] iii.twenty\text{°} [/latex] from the vertical because of the motorcar's acceleration. What is the magnitude of the acceleration of the car?

Show Solution

[latex] 0.548\,{\text{chiliad/s}}^{2} [/latex]

At a circus, a donkey pulls on a sled carrying a small clown with a force given past [latex] two.48\hat{i}+4.33\hat{j}\,\text{North} [/latex]. A horse pulls on the same sled, aiding the hapless donkey, with a strength of [latex] 6.56\hat{i}+5.33\hat{j}\,\text{N} [/latex]. The mass of the sled is 575 kg. Using [latex] \chapeau{i} [/latex] and [latex] \hat{j} [/latex] form for the reply to each trouble, detect (a) the cyberspace forcefulness on the sled when the two animals deed together, (b) the acceleration of the sled, and (c) the velocity after half dozen.50 s.

Hanging from the ceiling over a baby bed, well out of baby'due south reach, is a cord with plastic shapes, as shown here. The string is taut (there is no slack), as shown by the straight segments. Each plastic shape has the aforementioned mass thou, and they are every bit spaced by a distance d, as shown. The angles labeled [latex] \theta [/latex] depict the angle formed by the end of the string and the ceiling at each end. The center length of sting is horizontal. The remaining two segments each course an angle with the horizontal, labeled [latex] \varphi [/latex]. Let [latex] {T}_{ane} [/latex] be the tension in the leftmost section of the cord, [latex] {T}_{ii} [/latex] be the tension in the section next to it, and [latex] {T}_{3} [/latex] exist the tension in the horizontal segment. (a) Find an equation for the tension in each section of the string in terms of the variables m, g, and [latex] \theta [/latex]. (b) Detect the angle [latex] \varphi [/latex] in terms of the angle [latex] \theta [/latex]. (c) If [latex] \theta =5.10\text{°} [/latex], what is the value of [latex] \varphi [/latex]? (d) Notice the altitude 10 between the endpoints in terms of d and [latex] \theta [/latex].

Bear witness Solution

a. [latex] {T}_{1}=\frac{2mg}{\text{sin}\,\theta } [/latex], [latex] {T}_{2}=\frac{mg}{\text{sin}(\text{arctan}(\frac{1}{2}\text{tan}\,\theta ))} [/latex], [latex] {T}_{3}=\frac{2mg}{\text{tan}\,\theta }; [/latex] b. [latex] \varphi =\text{arctan}(\frac{1}{2}\text{tan}\,\theta ) [/latex]; c. [latex] 2.56\text{°} [/latex]; (d) [latex] 10=d(two\,\text{cos}\,\theta +2\,\text{cos}(\text{arctan}(\frac{1}{2}\text{tan}\,\theta ))+one) [/latex]

A bullet shot from a burglarize has mass of 10.0 g and travels to the right at 350 m/southward. It strikes a target, a large purse of sand, penetrating information technology a distance of 34.0 cm. Find the magnitude and direction of the retarding forcefulness that slows and stops the bullet.

An object is acted on by iii simultaneous forces: [latex] {\overset{\to }{F}}_{1}=(-iii.00\hat{i}+two.00\chapeau{j})\,\text{N} [/latex], [latex] {\overset{\to }{F}}_{two}=(6.00\hat{i}-4.00\hat{j})\,\text{N} [/latex], and [latex] {\overset{\to }{F}}_{3}=(2.00\hat{i}+5.00\hat{j})\,\text{Due north} [/latex]. The object experiences acceleration of [latex] iv.23\,{\text{g/s}}^{2} [/latex]. (a) Notice the acceleration vector in terms of m. (b) Find the mass of the object. (c) If the object begins from remainder, find its speed after 5.00 s. (d) Find the components of the velocity of the object subsequently 5.00 s.

Bear witness Solution

a. [latex] \overset{\to }{a}=(\frac{5.00}{yard}\hat{i}+\frac{3.00}{m}\hat{j})\,\text{thou}\text{/}{\text{s}}^{2}; [/latex] b. 1.38 kg; c. 21.two thou/s; d. [latex] \overset{\to }{five}=(xviii.1\chapeau{i}+10.9\hat{j})\,\text{one thousand}\text{/}{\text{s}}^{2} [/latex]

In a particle accelerator, a proton has mass [latex] 1.67\,×\,{ten}^{-27}\,\text{kg} [/latex] and an initial speed of [latex] 2.00\,×\,{10}^{5}\,\text{grand}\text{/}\text{south.} [/latex] Information technology moves in a directly line, and its speed increases to [latex] 9.00\,×\,{10}^{v}\,\text{m}\text{/}\text{s} [/latex] in a distance of 10.0 cm. Assume that the acceleration is constant. Find the magnitude of the forcefulness exerted on the proton.

A drone is being directed across a frictionless ice-covered lake. The mass of the drone is 1.50 kg, and its velocity is [latex] 3.00\hat{i}\text{m}\text{/}\text{s} [/latex]. After 10.0 s, the velocity is [latex] 9.00\hat{i}+iv.00\chapeau{j}\text{k}\text{/}\text{southward} [/latex]. If a constant force in the horizontal direction is causing this change in move, find (a) the components of the force and (b) the magnitude of the force.

Show Solution

a. [latex] 0.900\chapeau{i}+0.600\chapeau{j}\,\text{N} [/latex]; b. 1.08 N

crosbyhamosy02.blogspot.com

Source: https://courses.lumenlearning.com/suny-osuniversityphysics/chapter/5-7-drawing-free-body-diagrams/

Share this post